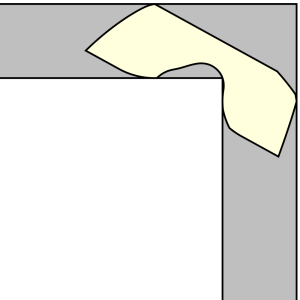
Modeling the situation of moving furniture around, the moving sofa problem asks for the maximum area of a planar shape that can move around the corner in an L-shaped hallway of width 1. The problem was posed by Leo Moser in 1966, and the best known lower bound of 2.2195... was proved by Gerver in 1994, by constructing a sofa whose boundary consists of 18 special curves. While it is conjectured that Gerver's sofa attains the maximum area, the best published upper bound of 2.37 was proved by Kallus and Romik in 2018 using computer assistance.

Improving upon the computer-assisted approach of Kallus and Romik, we improve the upper bound to 2.32. Moreover, without any computer assistance, we prove a conceptually new upper bound of 1 + \pi^2/8 = 2.2337... that is much closer to the lower bound of Gerver, on a large subset of shapes which includes Gerver's sofa. We also discuss the possibility of making the upper bound of 2.2337... unconditional by building upon the approaches of the two results.